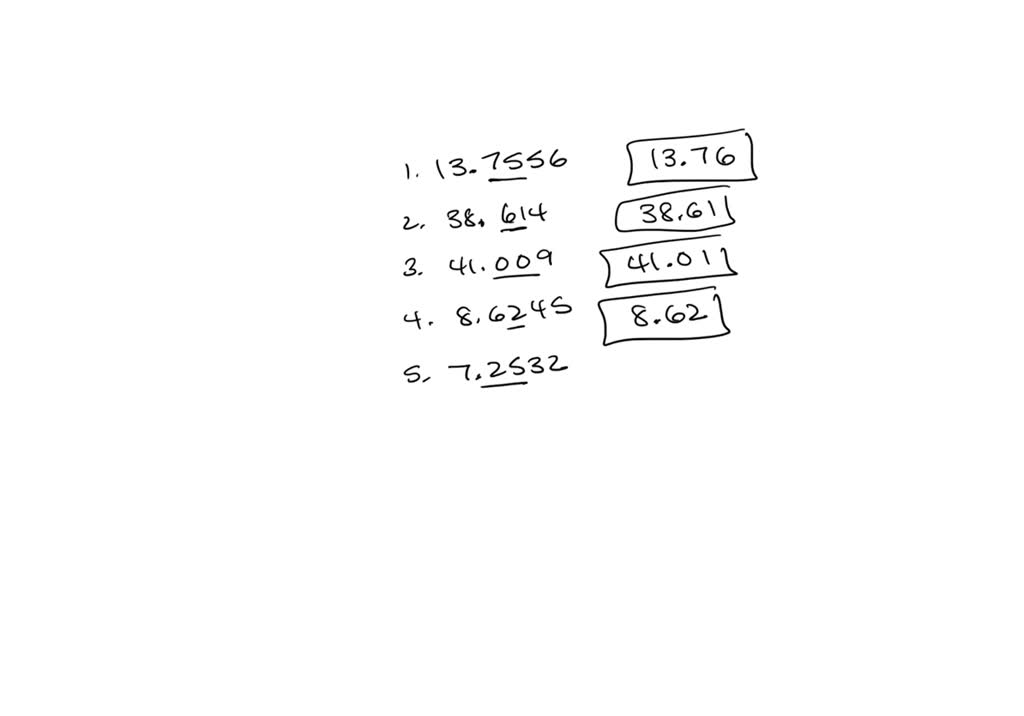Understanding how to round off to the nearest hundredths is an essential mathematical skill that has practical applications in various fields, from finance to engineering and everyday life. Whether you're a student, a professional, or simply someone who wants to improve their numerical abilities, mastering this concept is crucial. This article will delve deep into the process of rounding numbers to the nearest hundredths, providing clear explanations, examples, and practical tips to help you grasp this concept effortlessly.
Rounding numbers is not just about simplifying values; it's also about making data more manageable and easier to interpret. By understanding how to round off to the nearest hundredths, you can perform calculations more efficiently and communicate numerical information more effectively.
In this guide, we will explore the step-by-step process of rounding numbers, discuss common mistakes, and provide practical examples to ensure you fully understand the concept. Let's get started!
Read also:Vanessa Bryant Found True Love Again Meet Her New Husband
Table of Contents
- What is Rounding Off?
- Understanding Hundredths in Decimal Numbers
- Step-by-Step Process of Rounding Off to the Nearest Hundredths
- Practical Examples of Rounding Off to the Nearest Hundredths
- Common Mistakes to Avoid When Rounding Numbers
- Applications of Rounding Off in Real Life
- Mathematical Significance of Rounding Off
- Tools and Resources for Practicing Rounding Off
- Tips for Mastering Rounding Off
- Conclusion: Take Your Numerical Skills to the Next Level
What is Rounding Off?
Rounding off is a mathematical process used to simplify numbers while maintaining their approximate value. This technique is particularly useful when dealing with long decimal numbers or when exact precision is not necessary. By rounding off to the nearest hundredths, you can make calculations easier and present data in a more digestible format.
Why is Rounding Off Important?
Rounding off helps reduce the complexity of numbers, making them easier to work with. For instance, instead of dealing with a number like 3.14159, you can round it off to 3.14 for most practical purposes. This simplification is especially valuable in fields such as engineering, finance, and statistics, where large datasets need to be analyzed efficiently.
Understanding Hundredths in Decimal Numbers
In decimal numbers, the hundredths place is the second digit to the right of the decimal point. For example, in the number 0.56, the digit '6' represents the hundredths place. Understanding this position is crucial when rounding numbers to the nearest hundredths.
Identifying the Hundredths Place
To identify the hundredths place in a decimal number, follow these steps:
- Locate the decimal point in the number.
- Count two places to the right of the decimal point. The digit in this position is the hundredths place.
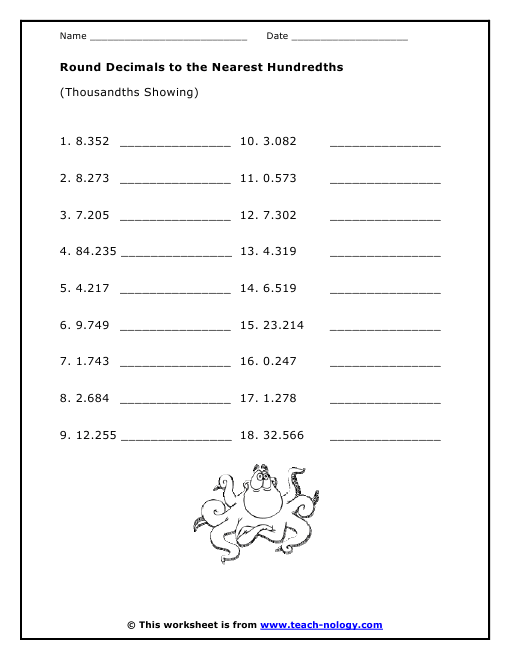
Step-by-Step Process of Rounding Off to the Nearest Hundredths
Rounding off to the nearest hundredths involves a systematic approach. Here's a step-by-step guide to help you master this process:
Step 1: Identify the Hundredths Place
As mentioned earlier, the hundredths place is the second digit to the right of the decimal point. For example, in the number 4.785, the hundredths place is '8'.
Read also:Katy Weaver The Rising Star In The Music Industry
Step 2: Look at the Digit in the Thousandths Place
The thousandths place is the third digit to the right of the decimal point. In the number 4.785, the thousandths place is '5'. This digit will determine whether you round up or down.
Step 3: Apply the Rounding Rule
If the digit in the thousandths place is 5 or greater, round up the hundredths place by one. If it is less than 5, leave the hundredths place unchanged. For example:
- 4.785 rounded to the nearest hundredths becomes 4.79.
- 4.784 rounded to the nearest hundredths becomes 4.78.
Practical Examples of Rounding Off to the Nearest Hundredths
Let's explore some practical examples to reinforce your understanding:
Example 1: Rounding 3.14159
The number 3.14159 has '4' in the hundredths place and '1' in the thousandths place. Since '1' is less than 5, the hundredths place remains unchanged. Therefore, 3.14159 rounded to the nearest hundredths is 3.14.
Example 2: Rounding 6.876
In the number 6.876, the hundredths place is '7' and the thousandths place is '6'. Since '6' is greater than 5, we round up the hundredths place by one. Thus, 6.876 rounded to the nearest hundredths is 6.88.
Common Mistakes to Avoid When Rounding Numbers
While rounding off seems straightforward, there are common pitfalls to watch out for:
Mistake 1: Forgetting to Check the Thousandths Place
Always ensure you check the digit in the thousandths place before making any rounding decisions. Ignoring this step can lead to incorrect results.
Mistake 2: Rounding Too Early
Perform all necessary calculations before rounding off. Rounding prematurely can introduce errors into your final result.
Applications of Rounding Off in Real Life
Rounding off to the nearest hundredths has numerous real-world applications, including:
Application 1: Financial Calculations
In finance, rounding off is often used to simplify monetary values. For example, when calculating interest rates or currency conversions, rounding to the nearest hundredths ensures precision without unnecessary complexity.
Application 2: Scientific Measurements
Scientists frequently use rounding to present data in a more understandable format. Whether it's measuring distances, temperatures, or chemical concentrations, rounding helps maintain clarity and accuracy.
Mathematical Significance of Rounding Off
Rounding off is not just a practical tool; it also holds mathematical significance. By approximating numbers, we can estimate solutions to complex problems more efficiently. Additionally, rounding helps reduce errors caused by excessive decimal places in calculations.
The Role of Rounding in Estimation
Estimation is a key component of problem-solving in mathematics. Rounding numbers to the nearest hundredths allows us to make quick and accurate estimations, saving time and effort in various scenarios.
Tools and Resources for Practicing Rounding Off
There are several tools and resources available to help you practice rounding off:
Tool 1: Online Rounding Calculators
Online rounding calculators provide instant results and are great for verifying your answers. Websites like Mathway and CalculatorSoup offer user-friendly interfaces for practicing rounding.
Resource 2: Educational Workbooks
Workbooks specifically designed for rounding off exercises can be invaluable for honing your skills. Look for materials that include step-by-step explanations and a variety of practice problems.
Tips for Mastering Rounding Off
Here are some tips to help you master the art of rounding off:
Tip 1: Practice Regularly
Like any skill, rounding off improves with practice. Set aside time each day to work on rounding exercises and gradually increase the complexity of the problems you solve.
Tip 2: Use Visual Aids
Visual aids such as number lines can help you better understand the concept of rounding. These tools provide a clear representation of how numbers are rounded up or down.
Conclusion: Take Your Numerical Skills to the Next Level
Rounding off to the nearest hundredths is a fundamental skill that has wide-ranging applications in both academic and professional settings. By following the step-by-step process outlined in this guide and practicing regularly, you can confidently tackle any rounding problem that comes your way.
We encourage you to share this article with others who might benefit from it and to explore more of our content for further learning opportunities. Remember, mastering rounding off is just the beginning—there's always more to discover in the world of mathematics!